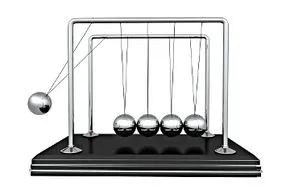
 In a Newton's Cradle, when a ball at one end strikes the others, it sends the ball on the opposite end soaring into the air. But why do the balls in the middle remain still? Zoonar/Thinkstock
In a Newton's Cradle, when a ball at one end strikes the others, it sends the ball on the opposite end soaring into the air. But why do the balls in the middle remain still? Zoonar/ThinkstockYou've likely encountered this device: Five small silver spheres suspended in a perfect line by thin threads, which attach to two parallel horizontal bars, all fixed to a base. These are common sights on office desks worldwide.
When you lift a ball and release it, it falls and strikes the others with a sharp click. Instead of all the remaining balls swinging outward, only the ball at the opposite end moves forward, while the others stay still. This ball gradually slows down, falls back, and briefly reunites with the rest before the cycle repeats.
This device is known as a Newton's Cradle, or sometimes a Newton's rocker or ball clicker. It was named in 1967 by English actor Simon Prebble, in tribute to his fellow countryman and groundbreaking physicist, Isaac Newton.
Though it appears simple at first glance, the Newton's Cradle, with its swinging and clicking balls, is far more than just a desk ornament. It serves as a refined display of some of the most foundational principles of physics and mechanics.
This device illustrates three key physics concepts: conservation of energy, conservation of momentum, and friction. In this article, we will explore these principles, delve into elastic and inelastic collisions, and examine both kinetic and potential energy. We will also reflect on the contributions of luminaries such as Rene Descartes, Christiaan Huygens, and the legendary Isaac Newton.
The History Behind the Newton's Cradle
 Sir Isaac Newton
Sir Isaac NewtonGiven that Isaac Newton was a pioneering figure in the development of modern physics and mechanics, it's only fitting that he would create a device like the Newton's Cradle, which so elegantly and simply demonstrates the fundamental laws of motion that he helped define.
He didn't, however.
The Newton's cradle, despite what its name suggests, was not actually invented by Isaac Newton, and the scientific principles behind it existed long before Newton's career in physics. In fact, it was John Wallis, Christopher Wren, and Christiaan Huygens who first presented theoretical papers to the Royal Society in 1662 that described the core principles at play in the device. Of these, Huygens was particularly notable for his recognition of momentum and kinetic energy conservation [source: Hutzler, etal]. Although Huygens didn’t use the term 'kinetic energy,' as it wouldn't be coined for another century, he did describe it as 'a quantity proportional to mass and velocity squared' [source: Hutzler, et al.].
French philosopher Rene Descartes (1596 - 1650) first proposed the concept of momentum, but his work was incomplete. His formula, momentum equals mass times speed (p=mv), worked in certain instances but failed in situations involving collisions between objects [source: Fowler].
It was Huygens who proposed modifying 'speed' to 'velocity' in the formula, which solved the issue. Unlike speed, velocity includes direction, so for two objects of equal size traveling at the same velocity in opposite directions, their combined momentum would total zero.
Even though Newton did not contribute to the scientific foundation of the cradle, he is credited with its name for two main reasons. First, the law of conservation of momentum can be derived from his second law of motion (force equals mass times acceleration, or F=ma). Ironically, Newton's laws of motion were published in 1687, 25 years after Huygens established the law of conservation of momentum. Secondly, Newton's far-reaching impact on the field of physics granted him greater fame than Huygens.
Newton's Cradle Design and Construction
A basic Newton's cradle setup consists of several balls suspended in a row from two horizontal bars, which are parallel to the line of the balls. These bars are securely mounted to a solid base to prevent any movement and ensure stability.
In the case of smaller cradles, the balls are suspended from the bars by fine wires, arranged in the shape of an inverted triangle. This alignment ensures the balls can only swing within a single plane, parallel to the crossbars. If they could swing in other planes, the energy transfer would be inefficient, and the device wouldn't function properly.
Ideally, all the balls should be identical in size, weight, mass, and density. While cradles with differently sized balls can still operate, they demonstrate the principles less effectively. The purpose of the cradle is to illustrate the conservation of energy and momentum, which both rely on mass. When one ball impacts another of equal mass, it transfers the same amount of energy and momentum, causing the second ball to move the same distance and speed. A larger ball, however, demands more energy to achieve the same movement, making the comparison less apparent.
As long as the balls are uniform in size and density, they can be as large or as small as desired. However, perfect alignment at the center is crucial for optimal performance. If the balls collide at an angle, energy and momentum are diverted, reducing efficiency. Cradles typically feature an odd number of balls, with five and seven being the most common, though any odd number will work.
Now that we understand how the balls are positioned, let's examine the materials they're made of and why these materials matter.
The Materials Used in Newton's Cradle Balls
In a Newton's Cradle, the balls are crafted from materials that are highly elastic and have consistent density. Elasticity refers to how well a material can stretch and return to its original shape without losing energy; the more elastic a material is, the less energy it dissipates. This is why a cradle with more elastic balls will continue moving for a longer time. The key takeaway is that the more something bounces, the higher its elasticity tends to be.
Stainless steel is frequently used for the balls in Newton's cradles because it is both highly elastic and relatively affordable. While metals like titanium are also elastic and could be effective, they tend to be much pricier.
Although it may appear that the balls in a Newton's cradle hardly deform when they collide, that's not entirely false. While a stainless steel ball might only compress a fraction of a millimeter upon impact, the cradle still operates efficiently because steel rebounds with minimal energy loss.
The balls' density must be uniform to ensure smooth energy transfer between them with minimal interference. A change in material density alters the way energy travels through it. For example, when vibration travels through air and steel, it travels further in steel due to its greater density, assuming the same amount of energy is applied initially. If a ball in a Newton's Cradle is denser on one side than the other, the energy transferred through the less-dense side may differ from what it received from the denser side, with the difference dissipated due to friction.
In Newton's cradles, especially those designed more for demonstration than aesthetics, other types of balls like billiard balls and bowling balls are commonly used. These balls are crafted from various types of hard resins that give them their strength and durability.
Amorphous metals represent a novel class of highly elastic alloys. During production, molten metal is cooled rapidly, causing the molecules to arrange randomly rather than forming crystalline structures like traditional metals. This molecular configuration makes them stronger than crystalline metals, as there are no pre-existing weak points. Amorphous metals would be excellent for use in Newton's cradles, though they are currently expensive to produce.
Principle of Energy Conservation
The law of conservation of energy asserts that energy, the capacity to do work, cannot be created or destroyed. However, it can change forms, which is exactly what happens in a Newton's Cradle—particularly with the conversion between potential energy and kinetic energy. Potential energy is stored energy, whether due to gravity or elasticity. Kinetic energy refers to the energy an object has due to its motion.
Let's label the balls from one to five. When all five are at rest, none of them have potential energy since they can't move any further downward, and they have no kinetic energy because they aren't in motion. When the first ball is lifted, it still has zero kinetic energy, but its potential energy increases due to gravity's ability to pull it down. Once released, the potential energy transforms into kinetic energy as the ball falls, thanks to the work done by gravity.
At the lowest point of its swing, the first ball's potential energy becomes zero, and its kinetic energy peaks. Since energy cannot be destroyed, the ball's maximum potential energy is equal to its maximum kinetic energy. When Ball One strikes Ball Two, it halts instantly, and both balls return to a state of zero energy. However, this energy doesn't disappear—it's transferred into Ball Two.
The energy from Ball One is transferred to Ball Two as potential energy, causing it to compress upon impact. As Ball Two decompresses and returns to its original shape, the potential energy converts into kinetic energy again, which is then passed on to Ball Three by compressing it. Essentially, the ball acts like a spring.
This energy transfer continues down the line, reaching Ball Five, the last ball. When Ball Five returns to its original shape, it doesn't have another ball to compress. Instead, its kinetic energy pushes Ball Four, causing Ball Five to swing outward. Thanks to the conservation of energy, Ball Five will have the same amount of kinetic energy as Ball One, meaning it will swing with the same speed as the first ball when it was released.
A single falling ball carries enough energy to move one ball the same distance and at the same speed as it fell. Similarly, two balls falling impart enough energy to move two balls, and this principle continues for all the balls in the line.
Why doesn't the ball simply bounce back to where it started? Why does the motion continue in just one direction? This is where momentum comes into play.
Momentum Conservation
Momentum refers to the force carried by moving objects; every object in motion has momentum, calculated by multiplying its mass by its velocity. Just like energy, momentum is conserved. It's crucial to understand that momentum is a vector quantity, meaning that the direction of motion is a key aspect of its definition. Simply stating that an object has momentum isn't enough—you must also indicate the direction in which that momentum is acting.
When Ball One strikes Ball Two, it moves in a specific direction, say from east to west. This means the momentum of Ball One is directed west as well. If the motion changes direction, the momentum must change, but this can only happen due to an external force. Therefore, Ball One doesn't just bounce off Ball Two—its momentum transfers energy through all the balls, pushing them in a westward direction.
But hold on. The ball pauses briefly at the top of its arc; if momentum requires continuous motion, how is it conserved in this moment? It might seem like the cradle is violating a fundamental law. The reason it isn't, however, is that the law of momentum conservation only applies within a closed system, one that is unaffected by external forces. The Newton's cradle isn't a closed system. As Ball Five swings away from the others, it also rises. During this rise, gravity acts on it, gradually slowing it down.
A more fitting example of a closed system would be pool balls: When the first ball strikes another, it stops while the second continues along a straight path, similar to how Newton's cradle balls would behave if not attached. (In reality, a truly closed system is unattainable because gravity and friction always play a role. However, in this analogy, gravity doesn't affect the motion, as it acts perpendicular to the balls' trajectory and does not influence their speed or direction.)
When the balls are at rest, forming a horizontal line, they function like a closed system, unaffected by any forces other than gravity. The brief interval between the impact of the first ball and the swing of the last ball is where momentum remains conserved.
At the top of its swing, the ball only holds potential energy, and its momentum and kinetic energy are both zero. Gravity then pulls it downward, kicking off the cycle once again.
Elastic Collisions and Friction
Two essential concepts come into play here, the first being elastic collisions. An elastic collision happens when two objects collide and the total kinetic energy remains unchanged before and after the impact. Imagine a Newton's cradle with just two balls: if Ball One has 10 joules of energy and strikes Ball Two in an elastic collision, Ball Two will swing away with the same 10 joules. The balls in a Newton's cradle collide elastically, transferring the energy of Ball One through the series of balls to Ball Five, with no energy loss in the process.
In a theoretical "ideal" Newton's cradle, only energy, momentum, and gravity would influence the balls, all collisions would be perfectly elastic, and the cradle's design would be flawless. In this ideal case, the balls would continue to swing indefinitely.
However, achieving an ideal Newton's cradle is impossible due to one unavoidable factor: friction. Friction gradually saps energy from the system, ultimately causing the balls to come to a halt.
While air resistance contributes a minor amount of friction, the primary source stems from within the balls themselves. Therefore, the interactions in a Newton's cradle aren't perfectly elastic collisions, but rather inelastic collisions, where the kinetic energy after the collision is less than it was before. This occurs because the balls are not perfectly elastic and cannot entirely avoid friction's effects. However, energy is still conserved. As the balls compress and return to their original shape, the friction between molecules converts kinetic energy into heat. Additionally, the balls vibrate, releasing energy into the air and generating the distinctive clicking sound of a Newton's cradle.
Flaws in the cradle's construction also contribute to the balls' slowing down. Misalignment or density differences among the balls will require more energy to move them. These imperfections deviate from the ideal Newton's cradle, leading to the eventual slowing of the balls at either end, causing them to swing together in unison.
For additional information on Newton's cradles, physics, materials, and related subjects, explore the following links.
